在MATLAB中计算复数共轭转置
矩阵的复数共轭转置是通过对原矩阵进行转置,然后对每个元素应用复数的复数共轭特性而得到的矩阵。在数学上,这也被称为矩阵的赫米特转置。
MATLAB提供了两种计算矩阵的复共轭转置的方法。
1.’ 操作符
2.ctranspose函数。
让我们通过例子来看看这两者的用法。
方法1:使用’ 操作符
语法:
vec_B = vec_A’
示例 1:
% MATLAB code for ' Operator
vecA = [1+2i 3+3.1i 4-1i;
2-0.1i -4i 0.4;
1i 0.23-1i 23+31i]; %matrix
% Displaying the original matrix
disp("Original Matrix")
disp(vecA)
% Computing the complex conjugate transpose of vecA
vecB = vecA';
% Displaying the complex conjugate transpose
disp("Complex Conjugate Transpose of vecA")
disp(vecB)
输出:

方法2:使用ctranspose()
ctranspose()函数与’运算符的工作相同,只是它能对类进行运算符重载。
% MATLAB code for ctranspose()
vecA = [1+2i 3+3.1i 4-1i;
2-0.1i -4i 0.4;
1i 0.23-1i 23+31i]; %matrix
% Displaying the original matrix
disp("Original Matrix")
disp(vecA)
% Computing the complex conjugate transpose of vecA
vecB = ctranspose(vecA);
% Displaying the complex conjugate transpose
disp("ctranspose of vecA")
disp(vecB)
输出:
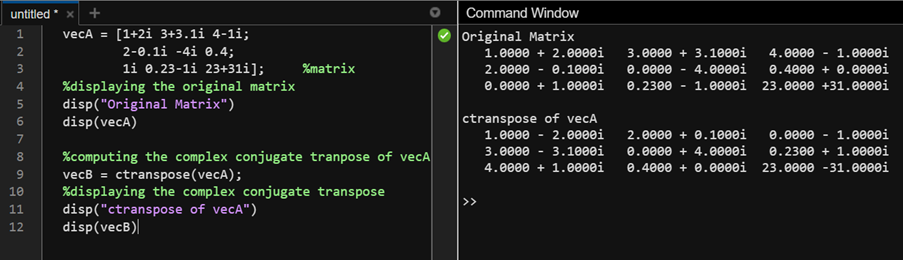
可以验证的是,两种方法的输出结果完全相同。
 极客教程
极客教程