在Python中根据极角对给定的笛卡尔点集进行排序的程序

假设我们有一个名为points的列表中含有一组笛卡尔点集。我们必须根据它们的极角来排序它们。极角的范围在0和2 * PI之间。如果某些点具有相同的极角，那么根据该点与原点的距离来排列它们。

因此，如果输入是points = [(1,1), (1,-2),(-2,2),(5,4),(4,5),(2,3),(-3,4)],

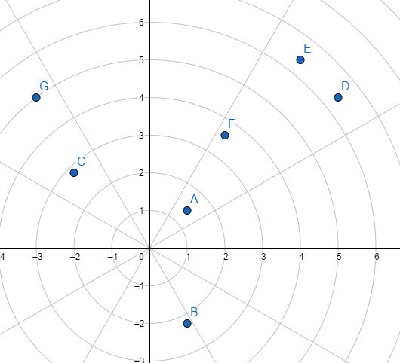
那么输出将是[(5, 4), (1, 1), (4, 5), (2, 3), (-3, 4), (-2, 2), (1, -2)]

为了解决这个问题，我们将采取以下步骤-

• 定义一个比较函数key()。这将取x
• atan：tan_inverse x[1]/x[0]
• 如果atan>= 0，则返回对(atan,x[1]^2+x[0]^2)否则返回(2*pi+atan,x[0]^2+x[1]^2)
• 然后使用比较函数key()对点进行排序

这里是一个例子

下面是实现的详细说明-

import math
def solve(points):
   def key(x):
      atan = math.atan2(x[1], x[0])
      return (atan, x[1]**2+x[0]**2) if atan >= 0 else (2*math.pi + atan, x[0]**2+x[1]**2)

   return sorted(points, key=key)

points = [(1,1), (1,-2),(-2,2),(5,4),(4,5),(2,3),(-3,4)]
print(solve(points))

输入

[(1,1), (1,-2),(-2,2),(5,4),(4,5),(2,3),(-3,4)]

输出

[(5, 4), (1, 1), (4, 5), (2, 3), (-3, 4), (-2, 2), (1, -2)]